Properties of Parallelogram
Properties of Parallelogram
PROPERTIES OF PARALLELOGRAM: A quadrilateral is a prallelogram if its both pairs of opposite sides are parallel to each other. In fig., quadrilateral ABCD is a prallelogram, because AB |  |
Theorem 1: A diagonal of a prallelogram divides it into two congruent triangles.
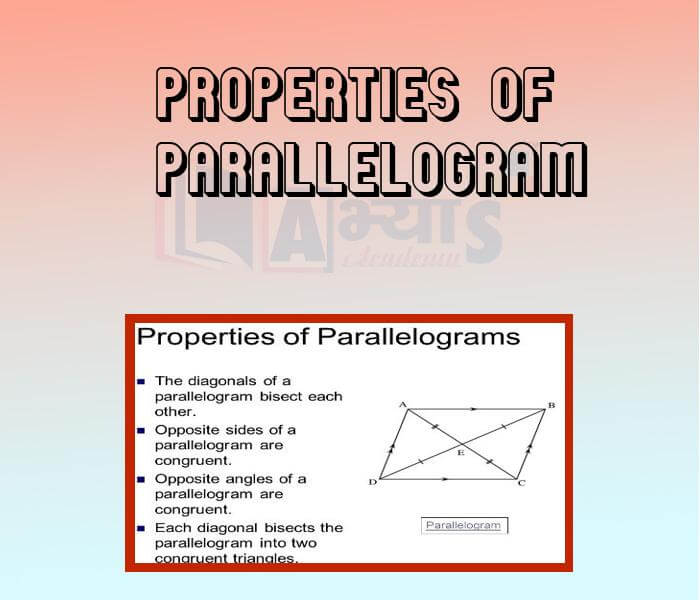
Theorem 2: In parallelogram, opposite sides are equal.
Theorem 3: The opposite angles of a parallelogram are equal.
Theorem 4: The diagonals of a parallelogram bisect each other.
Theorem 5: In a parallelogram, the bisectors of any two consecutive angles intersect at right angle.
Theorem 6: If diagonal of a parallelogram bisects one of the angles of the parallelogram, it also bisects the second angles. Also, prove that it is a rhombus.
Theorem 7: The angle bisectors of a parallelogram form a rectangle.
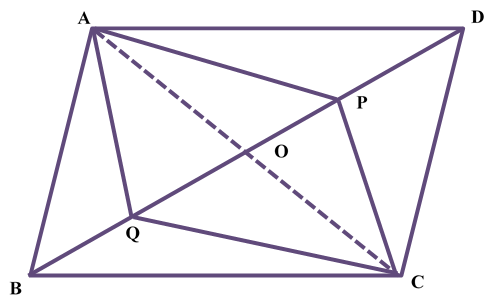
ILLUSTRATION: In a parallelogram ABCD two points P and Q are taken on the diagonal BD such that DP = BQ. Show that
(1) (2) AP = CQ (3)
(4) AQ= CP (5) APCQ is a parallelogram
Solution: In AD = CB [ opposite sides of a parallelogram] DP = QB [ Given] In AB = CD [ opposite sides of a parallelogram] BQ = DP [ Given] Now in the quadrilateral both the opposite pair of sides are equal.
|  |
The bisectors of angles of a parallelogram enclose a | |||
| Right Option : B | |||
| View Explanation |
Two adjacent angles of a parallelogram are equal. What is the measure of each of these angles? | |||
| Right Option : C | |||
| View Explanation | |||
In a parallelogram ABCD, if AB = 2x + 5, CD = y + 1 AD = y + 5 and BC = 3x - 4 then ratio of AB : BC is : | |||
| Right Option : C | |||
| View Explanation | |||
Students / Parents Reviews [10]
My experience with Abhyas is very good. I have learnt many things here like vedic maths and reasoning also. Teachers here first take our doubts and then there are assignments to verify our weak points.
Shivam Rana
7thMy experience was very good with Abhyas academy. I am studying here from 6th class and I am satisfied by its results in my life. I improved a lot here ahead of school syllabus.
Ayan Ghosh
8thAbhyas is a complete education Institute. Here extreme care is taken by teacher with the help of regular exam. Extra classes also conducted by the institute, if the student is weak.
Om Umang
10thIt has a great methodology. Students here can get analysis to their test quickly.We can learn easily through PPTs and the testing methods are good. We know that where we have to practice
Barkha Arora
10thIt was good as the experience because as we had come here we had been improved in a such envirnment created here.Extra is taught which is beneficial for future.
Eshan Arora
8thA marvelous experience with Abhyas. I am glad to share that my ward has achieved more than enough at the Ambala ABHYAS centre. Years have passed on and more and more he has gained. May the centre flourish and develop day by day by the grace of God.
Archit Segal
7thAbout Abhyas metholodology the teachers are very nice and hardworking toward students.The Centre Head Mrs Anu Sethi is also a brilliant teacher.Abhyas has taught me how to overcome problems and has always taken my doubts and suppoeted me.
Shreya Shrivastava
8thAbhyas Methodology is very good. It is based on according to student and each child manages accordingly to its properly. Methodology has improved the abilities of students to shine them in future.
Manish Kumar
10thBeing a parent, I saw my daughter improvement in her studies by seeing a good result in all day to day compititive exam TMO, NSO, IEO etc and as well as studies. I have got a fruitful result from my daughter.
Prisha Gupta
8thIt was a good experience with Abhyas Academy. I even faced problems in starting but slowly and steadily overcomed. Especially reasoning classes helped me a lot.
